Mathematics
Education
EMAT 6680,
Professor Wilson
Exploration Number 6, Triangle of Medians by
Ursula Kirk
1. Construct a triangle and its medians.
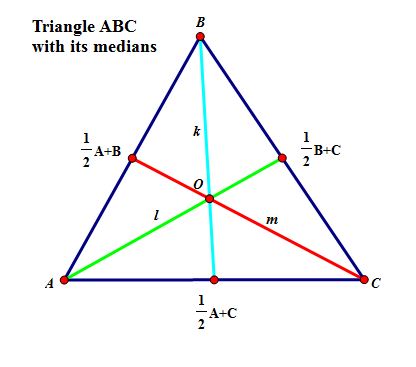
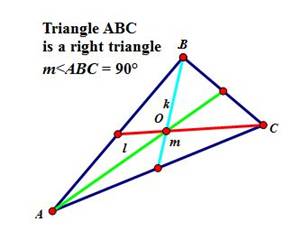
On Triangle ABC, we can see medians: k, l and m. These medians were be
found by constructing a segment from the vertex of the triangle to the midpoint
of the opposite side. The three medians of triangle ABC meet at point O, which
is the centroid of the triangle.
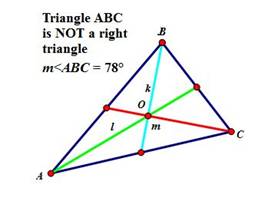
2. Construct a second triangle with the three sides
having the lengths of the three medians from your first triangle.
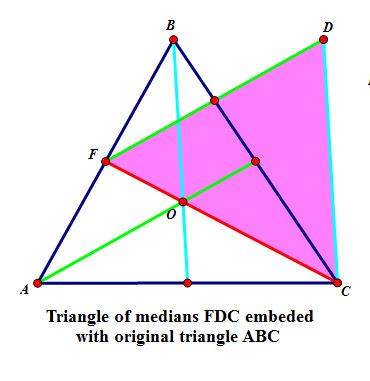
Here,
we have our original triangle ABC and a second triangle FDC made out the
medians of triangle ABC.
3. Find some
relationship between the two triangles.
·
Relationship
Number 1
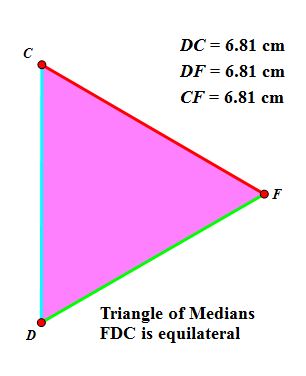
When triangle
ABC is equilateral, the triangle of medians FDC is also equilateral.
·
Relationship
Number 2
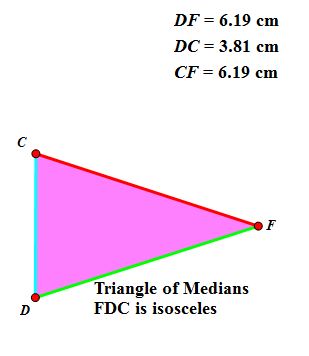
When
triangle ABC is isosceles, the triangle of medians FDC is also isosceles.
·
Relationship
Number 3
When
triangle ABC is a right triangle, the triangle of medians FDC is not always a
right triangle.
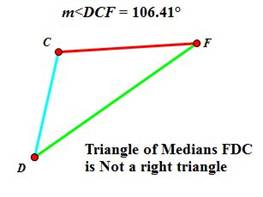
There is
one case where the resulting triangle FDC is a right triangle.
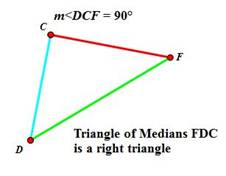
4. Show the area of the triangle of medians is 3/4 the area of the original triangle.
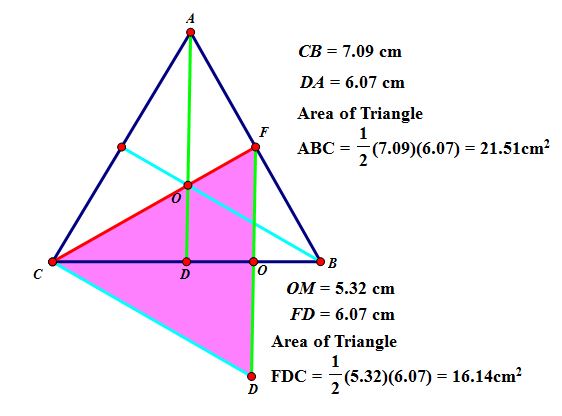
The area
of triangle ABC is 21.51 
and the area of triangle FDC is 16.14. We know that the area of triangle FDC has to be ¾ the
area of triangle ABC. Therefore:
Area FDC = 
5. Proof the area of the triangle of medians is 3/4 the area of the original triangle.
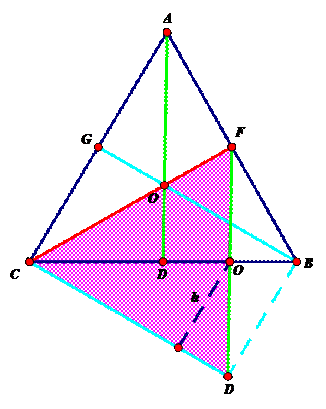
We must show that the
area of triangle CDF is 3/4 the area of triangle ABC. Since CDBG is a
parallelogram, then triangle CDB and triangle ACD have congruent areas.
Area of Triangle CDO = 
Area of Triangle CDB = 
Since OF || DA and OF
bisects AB then OF also bisects DB
Then BO = JF and
therefore OC = 
. Next we substitute for OC
The area of triangle CDO
= 
[ 
(BC) ] h
The area of triangle CDB
= (BC) h
Because of the definition
of medians, we know that the area of triangle ABC is twice the area of triangle
BDC
Area of triangle ABC = 2 [
(BC)] h
Area of triangle FCD is
twice the area of triangle CDO.
Area of triangle CDO = 2 [
* (BC) ] h
After cancelling out the
fractions:
Area of triangle CDF = [ BC * h ]
Area of triangle ABC = BC
* h
If those 2 formulas are
placed in a ratio the [BC * h] cancels out leaving us with the ratio of areas
of triangle CDF and ABC to be
*Adapted
from a proof by Robyn Bryant and Kaycie Maddox.